asebocities.blogg.se - Moment of inertia of a circle energy

#Moment of inertia of a circle energy how to

The moment of inertia about one end is 1 3 m L 2 1 3 m L 2, but the moment of inertia through the center of mass along its length is 1 12 m L 2 1 12 m L 2. This is because the axis of rotation is closer to the center of mass of the system in (b).

We see that the moment of inertia is greater in (a) than (b). Using the parallel-axis theorem eases the computation of the moment of inertia of compound objects. I total = I Rod + I Sphere = 1 3 m Rod L 2 + 2 5 m Sphere R 2 + m Sphere R 2 I total = I Rod + I Sphere = 1 3 m Rod L 2 + 2 5 m Sphere R 2 + m Sphere R 2 I Sphere = 2 5 m Sphere R 2 + m Sphere R 2 I Sphere = 2 5 m Sphere R 2 + m Sphere R 2.I total = I Rod + I Sphere = 1 3 m Rod L 2 + 2 5 m Sphere R 2 + m Sphere ( L + R ) 2 I total = I Rod + I Sphere = 1 3 m Rod L 2 + 2 5 m Sphere R 2 + m Sphere ( L + R ) 2

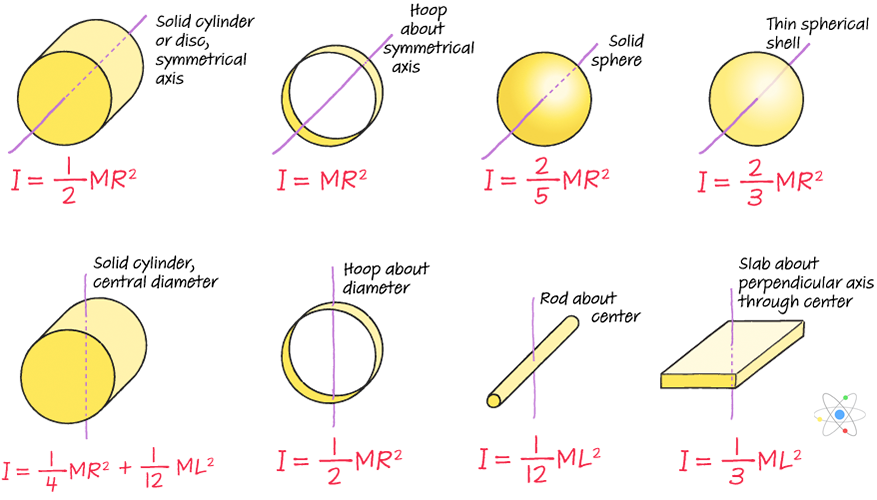
I Sphere = I center of mass + m Sphere ( L + R ) 2 = 2 5 m Sphere R 2 + m Sphere ( L + R ) 2 I Sphere = I center of mass + m Sphere ( L + R ) 2 = 2 5 m Sphere R 2 + m Sphere ( L + R ) 2

I total = ∑ i I i = I Rod + I Sphere I total = ∑ i I i = I Rod + I Sphere.

Refer to Table 10.4 for the moments of inertia for the individual objects. In both cases, the moment of inertia of the rod is about an axis at one end. In (b), the center of mass of the sphere is located a distance R from the axis of rotation. In (a), the center of mass of the sphere is located at a distance L + R L + R from the axis of rotation. Since we have a compound object in both cases, we can use the parallel-axis theorem to find the moment of inertia about each axis. The radius of the sphere is 20.0 cm and has mass 1.0 kg. The rod has length 0.5 m and mass 2.0 kg. Just as before, we obtainįind the moment of inertia of the rod and solid sphere combination about the two axes as shown below. The quantity dm is again defined to be a small element of mass making up the rod. We wish to ﬁnd the moment of inertia about this new axis ( Figure 10.26). Now consider the same uniform thin rod of mass M and length L, but this time we move the axis of rotation to the end of the rod. This happens because more mass is distributed farther from the axis of rotation. We would expect the moment of inertia to be smaller about an axis through the center of mass than the endpoint axis, just as it was for the barbell example at the start of this section.

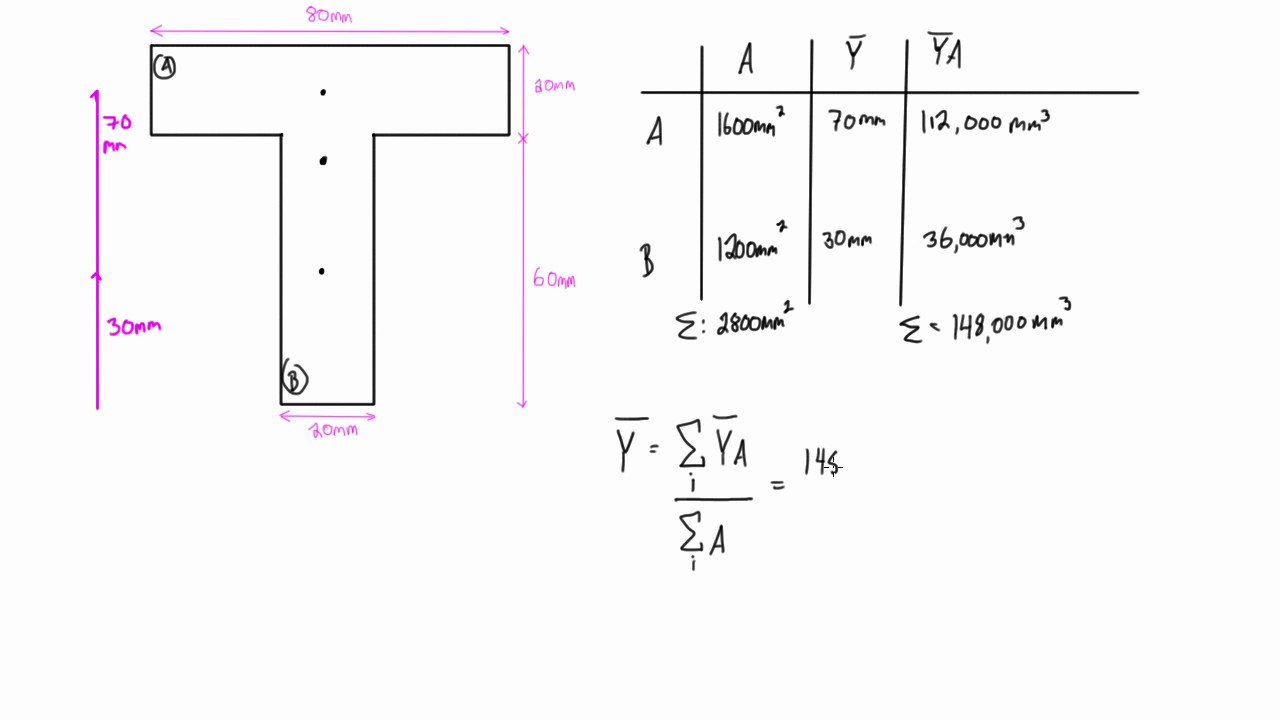
Next, we calculate the moment of inertia for the same uniform thin rod but with a different axis choice so we can compare the results. In the case with the axis in the center of the barbell, each of the two masses m is a distance R away from the axis, giving a moment of inertia of In this case, the summation over the masses is simple because the two masses at the end of the barbell can be approximated as point masses, and the sum therefore has only two terms. To see this, let’s take a simple example of two masses at the end of a massless (negligibly small mass) rod ( Figure 10.23) and calculate the moment of inertia about two different axes. Because r is the distance to the axis of rotation from each piece of mass that makes up the object, the moment of inertia for any object depends on the chosen axis. We defined the moment of inertia I of an object to be I = ∑ i m i r i 2 I = ∑ i m i r i 2 for all the point masses that make up the object.

#Moment of inertia of a circle energy how to

This section is very useful for seeing how to apply a general equation to complex objects (a skill that is critical for more advanced physics and engineering courses). In this section, we show how to calculate the moment of inertia for several standard types of objects, as well as how to use known moments of inertia to find the moment of inertia for a shifted axis or for a compound object. In the preceding section, we defined the moment of inertia but did not show how to calculate it. Calculate the moment of inertia for compound objects.Apply the parallel axis theorem to find the moment of inertia about any axis parallel to one already known.Calculate the moment of inertia for uniformly shaped, rigid bodies.By the end of this section, you will be able to: